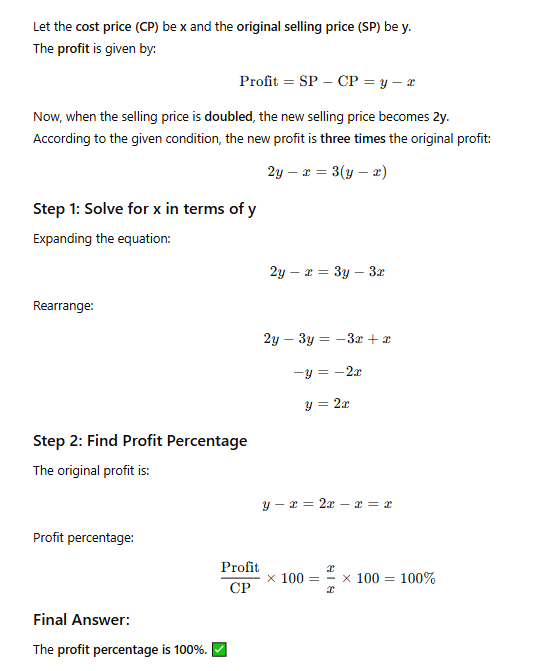
Another Methode

Let me help you understand this interesting profit problem by breaking it down carefully.

The key insight here is that the relationship between the selling price and profit gives us a way to find the original profit margin. Let’s think about this step by step.

First, let’s understand what we’re given. When the selling price doubles, the profit triples. This mathematical relationship actually tells us something important about the original prices. Let’s call the original cost price CP.

Now, let’s express the original selling price (SP) in terms of CP: Original SP = CP + Profit If we call the original profit ‘p’, then: Original SP = CP + p

When the selling price doubles: New SP = 2(CP + p) New SP = 2CP + 2p

Since this doubled selling price results in a tripled profit: New Profit = 3p We can also write the new profit as: New SP – CP = 3p Therefore: 2CP + 2p – CP = 3p Simplifying: CP + 2p = 3p Further simplifying: CP = p

This is a crucial realization! The original profit equals the cost price. This means the original selling price was exactly twice the cost price.

Now we can easily calculate the profit percentage: Profit Percentage = (Profit ÷ CP) × 100 Since profit equals cost price: = (CP ÷ CP) × 100 = 100%

Therefore, when the selling price is doubled and the profit triples, the original profit percentage must have been 100%.

To verify this makes sense:

• If CP = x, then original profit = x (100%)
• Original SP = 2x
• When SP doubles: New SP = 4x
• New profit = 3x (triple the original profit)
• Original CP remains x

This elegant solution shows us that for this relationship to hold true, the initial markup had to be 100%. This kind of problem helps us understand how mathematical relationships in pricing can reveal underlying business metrics.