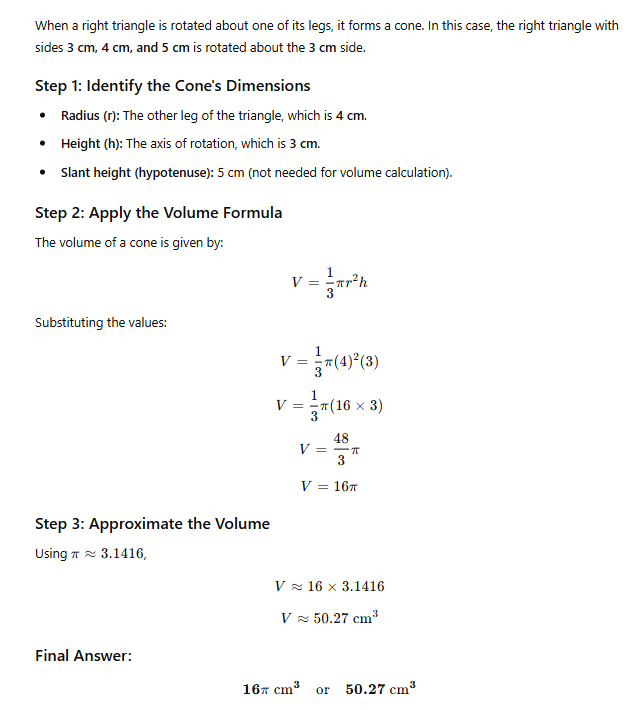
A right triangle with sides 3 cm, 4 cm and 5 cm is rotated the side of 3 cm to form a cone. The volume of the cone so formed is:
Step 1: Understanding the Rotation
The given right triangle has:
- Base = 4 cm
- Height = 3 cm
- Hypotenuse = 5 cm (not needed for volume)
When this triangle is rotated about the 3 cm side, it forms a cone where:
- The radius (r) = 4 cm (the other leg)
- The height (h) = 3 cm (the axis of rotation)
Step 2: Using the Volume of Revolution (Calculus Method)
We can determine the volume using the disk method, but in this case, it is simpler to use the standard volume formula of a cone:
V=13πr2hV = \frac{1}{3} \pi r^2 hV=31πr2h
Step 3: Plugging in the Values
V=13π(4)2(3)V = \frac{1}{3} \pi (4)^2 (3)V=31π(4)2(3) V=13π(16×3)V = \frac{1}{3} \pi (16 \times 3)V=31π(16×3) V=483πV = \frac{48}{3} \piV=348π V=16πV = 16\piV=16π
Step 4: Approximation (if needed)
Using π≈3.1416\pi \approx 3.1416π≈3.1416:
V≈16×3.1416V \approx 16 \times 3.1416V≈16×3.1416 V≈50.27 cm3V \approx 50.27 \text{ cm}^3V≈50.27 cm3
Conclusion:
Using either geometric reasoning or calculus concepts, the volume of the cone remains:
16π cm3or50.27 cm3\mathbf{16\pi \text{ cm}^3} \quad \text{or} \quad \mathbf{50.27 \text{ cm}^3}16π cm3or50.27 cm3
